About Critical Ratio Calculator (Formula)
The Critical Ratio Calculator is a statistical tool used to compare two sample means to determine if the difference between them is significant. This calculation is essential in many fields, including research, business, and education, where data comparison is crucial. By using a critical ratio, you can assess whether observed differences are likely due to chance or a genuine effect. It helps in hypothesis testing and is valuable for making informed decisions based on data.
Formula
The formula to calculate the critical ratio is:
Critical Ratio = (Mean 1 – Mean 2) divided by the square root of (SD 1 squared divided by N 1 plus SD 2 squared divided by N 2).
In this formula:
- Mean 1 and Mean 2 represent the averages of the two groups or samples.
- SD 1 and SD 2 are the standard deviations of the two samples.
- N 1 and N 2 are the sizes of the two samples.
How to Use
- Gather Data: Obtain the mean, standard deviation, and sample size for both groups or sets of data.
- Apply the Formula: Subtract Mean 2 from Mean 1. Then, square the standard deviations and divide them by the respective sample sizes. Add the results and take the square root.
- Interpret the Result: The critical ratio tells you how many standard deviations the difference between the means is away from zero. A higher absolute value indicates a more significant difference.
Example
Suppose you are comparing the test scores of two different groups of students.
- Group 1 has a mean score of 85, a standard deviation of 5, and a sample size of 30.
- Group 2 has a mean score of 80, a standard deviation of 4, and a sample size of 25.
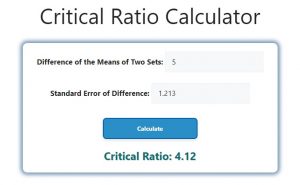
First, calculate the difference in means:
85 – 80 = 5
Next, calculate the variance components:
For Group 1: (5² / 30) = 25 / 30 = 0.833
For Group 2: (4² / 25) = 16 / 25 = 0.64
Now, add these values and take the square root:
√(0.833 + 0.64) = √1.473 = 1.213
Finally, calculate the critical ratio:
5 / 1.213 = 4.12
A critical ratio of 4.12 suggests a significant difference between the two groups’ scores.
FAQs
1. What is a Critical Ratio Calculator?
A Critical Ratio Calculator is a tool that helps compare the means of two groups to see if their difference is statistically significant.
2. What does the critical ratio measure?
The critical ratio measures how far apart the means of two groups are in terms of standard deviations, helping to determine if the difference is meaningful.
3. What is considered a significant critical ratio?
A critical ratio above 2 or below -2 generally indicates a statistically significant difference, depending on the context.
4. Can I use this calculator for small sample sizes?
Yes, but smaller sample sizes can lead to less reliable results, so it’s essential to interpret the critical ratio carefully.
5. How is the critical ratio related to hypothesis testing?
In hypothesis testing, the critical ratio helps determine whether to reject the null hypothesis, indicating that there is no significant difference between the groups.
6. Do both groups need to have the same sample size?
No, the sample sizes (N 1 and N 2) can differ, and the formula accounts for this variation.
7. How does standard deviation affect the critical ratio?
A higher standard deviation increases the denominator in the critical ratio formula, which can lower the critical ratio and reduce the likelihood of finding significance.
8. Is the critical ratio the same as a t-test?
The critical ratio is similar to a t-test but is often used in contexts where the t-distribution may not apply or when comparing small sample sizes.
9. Can I use the critical ratio for comparing more than two groups?
No, the critical ratio is designed to compare only two groups at a time. For more than two groups, you would need other statistical tests like ANOVA.
10. What is the difference between a critical ratio and a z-score?
The critical ratio is a specific application of the z-score formula, used for comparing the means of two groups rather than individual data points.
11. How does this calculator help in research?
The Critical Ratio Calculator helps researchers determine if differences between groups in experiments are statistically significant, aiding in data interpretation.
12. Is it important to have equal variances between groups?
Ideally, groups should have similar variances, but the critical ratio formula can still be used if variances differ, though it might affect interpretation.
13. Can the calculator be used for business data analysis?
Yes, businesses can use the Critical Ratio Calculator to compare sales figures, customer satisfaction scores, or other data to make informed decisions.
14. What role does sample size play in the calculation?
Larger sample sizes reduce the variability of the critical ratio, making it more likely to detect significant differences between groups.
15. How do I interpret a critical ratio close to 0?
A critical ratio near 0 indicates that the means of the two groups are very similar, and the difference is likely not significant.
16. What if my critical ratio is negative?
A negative critical ratio means that the second group’s mean is higher than the first group’s, but the magnitude of the ratio determines significance.
17. Can I calculate the critical ratio by hand?
Yes, the formula is straightforward, but using a calculator saves time and reduces the risk of errors.
18. How does the calculator handle decimal points?
The calculator handles decimals in the means, standard deviations, and sample sizes to provide an accurate critical ratio.
19. Can I use this calculator for non-numeric data?
No, the Critical Ratio Calculator is designed for numeric data, particularly means and standard deviations from quantitative datasets.
20. What should I do if my critical ratio is not significant?
If the critical ratio is not significant, the difference between the groups may be due to random variation, and you may not need to act on the data.
Conclusion
The Critical Ratio Calculator is a valuable tool for anyone involved in data analysis. Whether you’re a researcher, business analyst, or educator, understanding the critical ratio helps you determine the significance of differences between two groups. By applying this calculation, you can make data-driven decisions with confidence, knowing whether observed variations are likely meaningful or just due to chance.